Lattice Enthalpy
- Standard lattice enthalpy of formation, ΔLEH⦵ is the enthalpy change when one mole of an ionic lattice is formed from its gaseous ions under standard conditions.
- Standard lattice enthalpy of dissociation is the enthalpy change when one mole of an ionic lattice completely dissociates into its gaseous ions under standard conditions.
- The enthalpy of formation and the enthalpy of dissociation are opposites.
- Lattice enthalpy gives an indication of the strength of the ionic bonds
- Lattice enthalpy cannot be measured directly, so other measurements need to be known
- Standard Enthalpy of Formation, ΔfH⦵ is the enthalpy change when one mole of a compound is formed from its constituent elements in their standard states, under standard conditions
- Standard Enthalpy of Atomisation, ΔaH⦵ is the enthalpy change when one mole of gaseous atoms are formed from an element in its standard state.
- First ionisation energy,ΔI1H⦵ is the energy required to remove one mole of electrons from one mole of gaseous atoms to form one mole of gaseous ions.
- Second ionisation energy, ΔI2H⦵ is the enthalpy change when one mole of gaseous ions are formed from one mole of gaseous ions
- First electron affinity, ΔEA1H⦵ is the enthalpy change when one mole of gaseous 2^+ ions are formed from one mole 1^+ of gaseous atoms
- Second electron affinity, ΔEA2H⦵ is the enthalpy change when one mole of gaseous 2^- ions are formed from one mole of gaseous 1^- ions
- The first electron affinity is always exothermic because the electron is attracted to the positively charged nucleus of an atom. But the second electron affinity is endothermic because energy must be put in to overcome the repulsion between the electron and negatively charged ion
 |  |
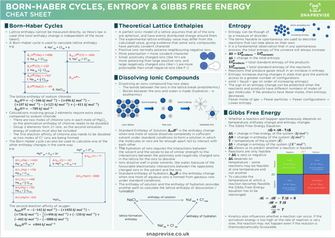
Born-Haber Cycles
- Lattice enthalpy cannot be measured directly, so Hess’s law is used (the total enthalpy change is independent of the route taken).
- A Born-Haber cycle is used to calculate lattice enthalpy
- Calculations involving group 2 elements require extra steps compared to sodium chloride:
- There are two moles of chlorine ions in each mole of MgCl2, so the atomisation enthalpy of chlorine needs to be doubled
- Group 2 elements form 2+ ions, so the second ionisation energy of sodium must also be included
- The first electron affinity of chlorine also needs to be doubled as two moles of Cl^- ions are being formed
- The Born-Haber cycle can also be used to calculate one of the other enthalpy changes in the same way
Theoretical Lattice Enthalpies
- A perfect ionic model of a lattice assumes that all of the ions are spherical, and have evenly distributed charge around them
- The experimental lattice enthalpy value may differ from the calculated value, giving evidence that some ionic compounds have partially covalent character
- Positive ions normally polarise neighbouring negative ions
- More polarisation = more covalent character
- Small, positively charged ions (like H+) are more polarising than large positive ions, and large negatively charged ions (like I-) are more polarisable than small negative ions (like F-)
Dissolving Ionic Compounds
- Dissolving an ionic compound has two steps
- The bonds between the ions in the lattice break (endothermic)
- Bonds between the ions and water is made (hydration- is exothermic)
- Standard Enthalpy of Solution, ΔsolH⦵ is the enthalpy change when one mole of solute dissolves completely in sufficient solvent under standard conditions to form a solution in which the molecules or ions are far enough apart not to interact with each other
- The hydration of ions requires the interactions between the solvent and the solute to be of similar strength to the interactions between the positively and negatively charged ions in the lattice for the ions to dissolve
- Ions dissolve well in polar solvents, like water, because of the favourable electrostatic interactions between the oppositely charged ions in the solvent and the ions
- Standard enthalpy of hydration, ΔhydH is the enthalpy change when one mole of aqueous ions is formed from gaseous ions under standard conditions.
- The enthalpy of solution and the enthalpy of hydration provides another path to calculate the lattice enthalpy of dissociation / formation
Entropy
- Entropy can be though of as a measure of disorder
- The terms feasible or spontaneous are used to describe reactions that can take place on their own.
- It is a fundamental observation that in any spontaneous process, the total entropy of the universe will always increase
Reactions that produce gases result in an increase in entropy
- Entropy increases during changes in state that give the particle access to a greater number of configurations
- The sign of an entropy change can be predicted when the reactants and products have different numbers of moles of gas molecules. If the products have fewer moles, then entropy decreases
Gibbs Free Energy
- Whether a reaction will happen spontaneously depends on temperature, enthalpy change and entropy changes
- The Gibbs Free-Energy Equation:
ΔG = change in free energy of the system (kJ mol^-1)
∆H = change in enthalpy of the system (kJ mol^-1)
T = temperature of the system (K)
∆S = change in entropy of the system (J K^-1 mol^-1)
- ΔG allows us to predict whether a reaction is feasible
- Reactions are only feasible if ΔG is zero or negative
- ΔG depends on temperature – some reactions may be feasible at one temperature and not another
- To calculate the temperature at which a reaction becomes feasible, the Gibbs Free-Energy equation has to be rearranged
- Kinetics also influences whether a reaction can occur. If the activation energy is too high or the rate of reaction is very slow, the reaction may not happen even if the reaction is thermodynamically favourable.